In this article presents logarithmic methods for solving first order and second order differential equations.
Let 
, 
be Riemann integrable functions; 
, 
, 
, 
; 
, 
, 
, … , 
, 
, 
- is an integration constant. The symbol 
between two formulas will mean that the second formula follows from the first one.
1. First order differential equations
1.1. Linear inhomogeneous first order differential equation [1], [2], [3]:
. (1.1)
Logarithmic integration method. In equation (1.1) the function is not identically zero. Then 
be not identically zero. Then with equation (1.1), consequently we get
,
,
, (1.2)
,
,
,
,
,
,
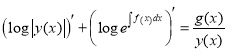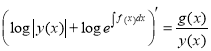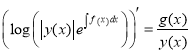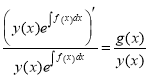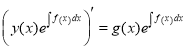. (1.3)
Remark 1.1. A similar method can be used to obtain a solution of the equation (1.1) in the Cauchy form [3]:
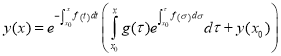, (1.4)
where 
is a given constant.
Indeed, the equation (1.2) is equivalent to the equation
, (1.5)
where
is an integration constant. Let 
, where 
. Then the equation (1.5) can be represented as
,
,
,
,
,
,
,
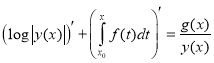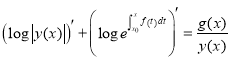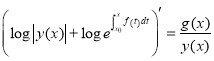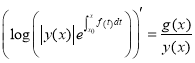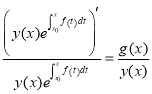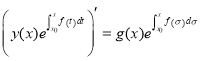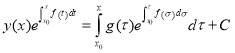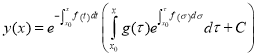. (1.6)
If in the equation (1.6) we let 
, then we have the formula (1.4).
1.2. Bernoulli differential equation [3]:
, (1.7)
where 
.
Logarithmic integration method. Let 
be not identically zero. Then from the equations (1.7) we obtain
,
,
,
,
,
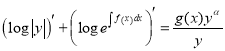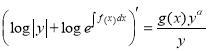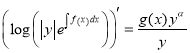, (1.8)
,
,
,
,
,
,
,
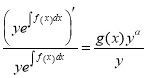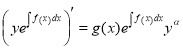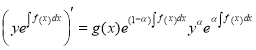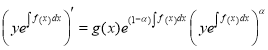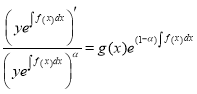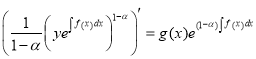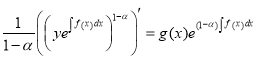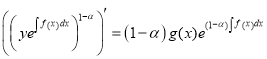, (1.9)
,
,
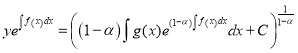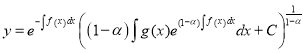. (1.10)
Remark 1.2. At the beginning of the course of the method, we assumed that be not identically zero. It follows that the equation (1.7) has a particular solution 
, if 
.
Remark 1.2.1.(The second version of the logarithmic method.) In the equations (1.7) we obtain
,
,
,
,
,
,
,
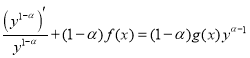. (1.11)
The equation (1.11) is a linear inhomogeneous first order differential equation, with respect to the function 
. Its solution by the with formula (1.3), has the form
. (1.12)
The formula (1.12) implies the solution (1.10).
Remark 1.2.2.(The third version of the logarithmic method.) In the equations (1.8) we obtain
,
,
,
,
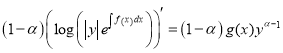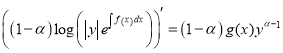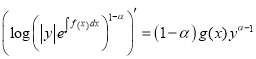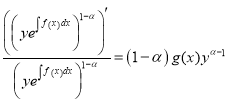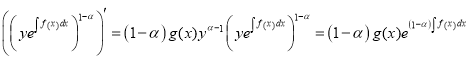. (1.13)
The equation (1.13) is similar to the equation (1.9).
1.3. The equation of the form:
, (1.14)
where 
.
Logarithmic integration method. From the equation (1.14), we obtain
,
,
,
,
,
,
,
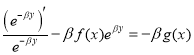. (1.15)
The equation (1.15) is a linear inhomogeneous first order differential equation, with respect to the function 
. Its solution, by the formula (1.3), has the form
. (1.16)
Solving the equation (1.16), with respect to 
, we have
,
. (1.17)
Second order differential equation
2.1. Linear homogeneous second order differential equation [1], [3]:
, (2.1)
where 
, 
are real numbers.
Let 
be not identically zero. Then from the equations (2.1) we obtain
,
, (2.2)
because 
, 
,
.
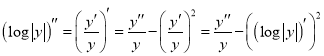Let in the equation (2.2):
.
Then we have equation (2.2) in the form
, (2.3)
. (2.4)
Case 1. 
. In this case we have equation (2.4) has be form
,
,
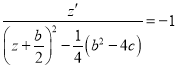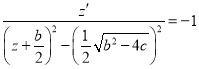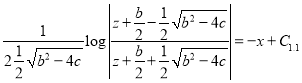. (2.5)
Let in the equation (2.5): 
, 
. Then we obtain
,
, 
,
,
, 
,
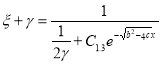. (2.6)
Returning to the change of variables , , in the equation (2.6), we obtain
,
,
, 
,
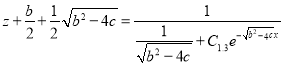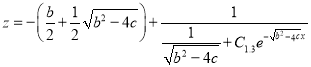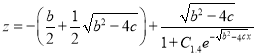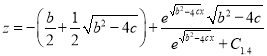. (2.7)
Since 
, then we have in the equation (2.7)
,
,
,
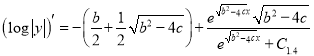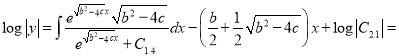
, (2.8)
where 
, 
is an integration constant.
Case 2. 
. In this case we have equation (2.4) has be form
.
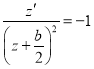Step by step from the last equation we obtain
,
, 
,
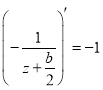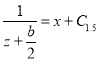. (2.9)
Since
, then we have in the equation (2.9)
,
,
, (2.10)
where 
is an integration constant.
Case 3. 
. In this case we have equation (2.4) has be form
,
,
,
, 
,
,
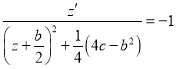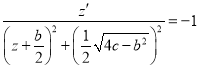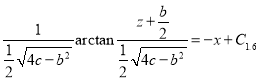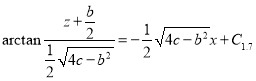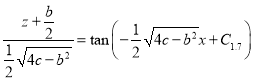. (2.11)
Since , then we have in the equation (2.11)
,
,
, (2.12)
where 
, 
is an integration constant.
The formulas (2.8), (2.10), (2.12) solve the equation (2.1) in the respective cases 1,2,3. This method makes it possible to obtain these solutions without applying a complex analysis and finding a solution in the form 
.
References:
- C. H. Edwards, D. E. Penny. Differential Equations and Boundary Value Problems: Computing and Modeling (Third Edition), (2010) — 708 p.
2. C. H. Edwards, D. E. Penny, D. Calvis. Elementary differential equations, — 632 p.
3. N. M. Matveev. Metodu integrirovaniya obiknovennih differentsialnih uravneniy, Izdatyelstvo leningradskoho universiteta, (1955) — 655 p.
